| Versão em Português |
Kepler's Laws
Worksheets demonstrating Kepler's Laws of Planetary Motion.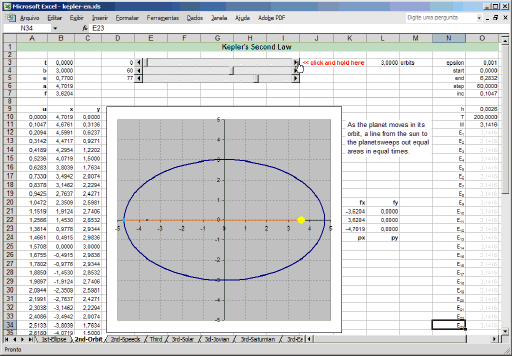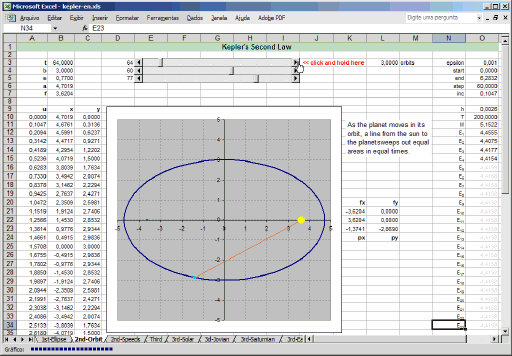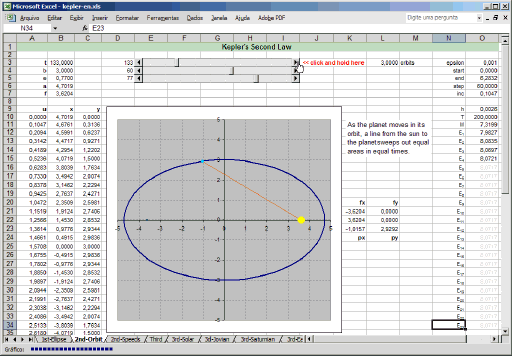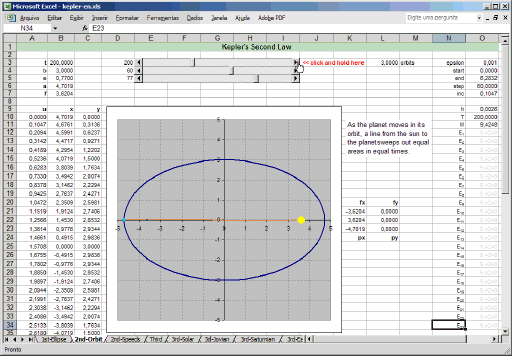
Click on the image to download the workbook
Kepler's First and Second Laws
The first two worksheets have animated graphics demonstrating the elementary ellipse parameters and -- my favorite -- a planet moving around the ellipse, getting faster as it draws closer to the sun and slower as the distance increases. The worksheet solves Kepler's equation using the same iterative method Kepler himself used nearly 400 years ago. But he did it by hand. I think it's funny that people still teach Kepler's 2nd law with almost the exact same words as he originally phrased it. The "sweeps out equal areas in equal times" thing is because the mathematics in Kepler's time was mostly geometry-based, so he tended to think in terms of lengths, areas. It was a geometer's way to parametrize the change of speed of the planet as a function of time -- that's why it sounds odd. Our modern analytic geometry makes it so much easier to understand.Kepler's Third Law
Did you ever wonder how astronomers know how much the planets weigh? If you plot the average distance of each planet's orbit around their central body (e.g., the sun) versus its orbital period in log-log scale, you'll get a nice straight line with inclination 2/3. This is, in fact, a modern way to formulate Kepler's Third Law of Planetary Motion. The original version, as he wrote in 1619 in his book Harmonices Mundi, reads something like: "The squares of the periodic times are to each other as the cubes of the mean distances". Or, more concisely, T2=kR3, where T is the planet's orbital period, R is is mean distance from the central body and k is a proportionality constant. It took Kepler (1571-1630) nearly his whole life to achieve this result; nowadays we can reproduce it in an Excel worksheet in a few minutes. Isaac Newton later found out, when developing his theory of gravitation, that k is in fact GM/4π2, where M is the mass of the central body and the mass of the planet is negligible compared with M. In other words, Kepler's third law can be used calculate the mass of the central body. That's how astronomers know the mass of the sun and other planets that have satellites. The last worksheets in the workbook have log-log plots of the periods and distances for the planets in the solar system, the jovian system (Jupiter and its satellites) and the saturnian system. You can verify that the inclination of the line is very close to the value of 2/3 predicted by theory. The y-intercept is used to compute k, which, in turn, is used to calculate the mass of each central body.To Dos
The current version is 2D-only. I'd like to move to 3D.- Make a 3D version of the Orbits sheet, showing the geometry of the situation as astronomers define.
- Make a 3D version with two planets and the radius vector from one to the other
- Make a "planetarium"-style sheet, showing the apparent motion of a planet as seen from the other
- Make a sheet with a comet in a parabolic orbit
- Make a sheet with a comet in hyperbolic orbit
